Publications
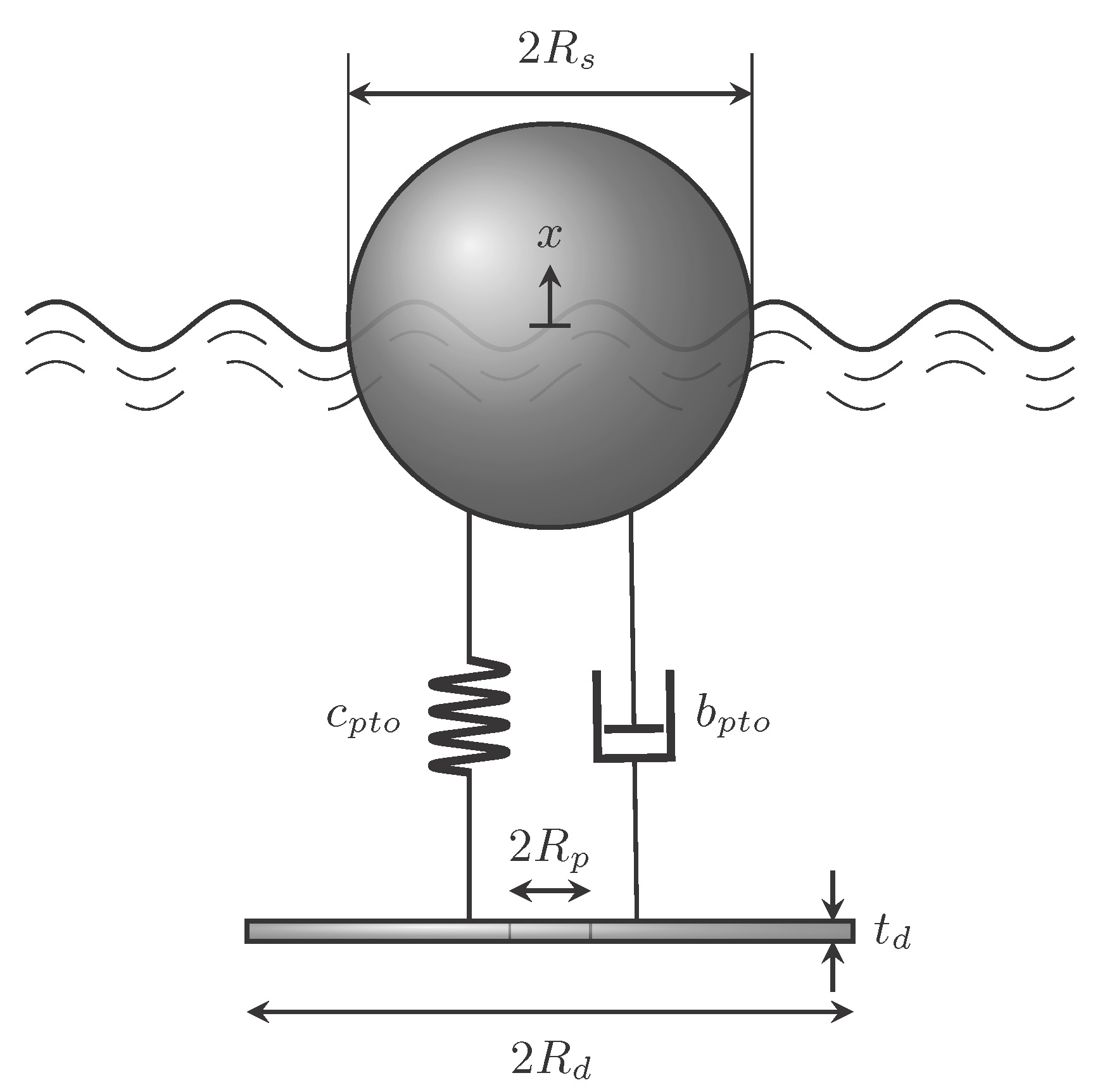
43. Performance of a two-body wave energy converter with an annular heave plate
R. Tamakuwala, M. Usman, N. Tom, and H. Masoud, Flow 5, E32 (2025) pdf
We theoretically examine the performance of a two-body wave energy converter (WEC) featuring a floating sphere and a submerged annular heave plate, connected by a power take-off (PTO) system. Utilising linear wave theory, we derive the system’s frequency-domain response to regular plane waves and analyse the impact of varying disk porosity on power generation. Our results suggest that annular disks can enhance power extraction efficiency in various cases compared with solid heave plates. Additionally, permeable plates can broaden operational conditions by reducing oscillation amplitudes and decreasing the mechanical strain on the PTO system without substantially compromising the power conversion efficiency. Overall, our findings provide valuable insights for optimising WEC designs to improve energy capture, emphasising the potential hydrodynamic advantages of using porous reaction bodies.

42. Small-amplitude heave oscillations of an annular disk
M. Usman and H. Masoud, Journal of Fluid Mechanics 1017, A21 (2025) pdf
We theoretically investigate the small-amplitude broadside oscillations of an annular disk within an unbounded fluid domain. Specifically, we formulate a semi-analytical framework to examine the effects of the oscillation frequency and pore radius on the disk’s added mass and damping coefficients. By leveraging the superposition principle, we decompose the complex original problem into two simpler ones. The force exerted on the disk by the fluid is linked to the solutions of these sub-problems through the reciprocal theorem; the first solution is readily available, while the second is derived asymptotically, assuming a small inner radius. Both solutions are evaluated by transforming dual integral equations into systems of algebraic equations, which are then solved numerically. Building on these solutions, we extract asymptotic expressions for the variations of the quantities of interest in the limits of low and high oscillatory Reynolds numbers. Notably, at high frequencies, we uncover a previously overlooked logarithmic term in the force coefficient expansions, absent in prior scaling analyses of oscillating solid (impermeable) disks. Our findings indicate that, when viscosity plays a dominant role, an annular (porous) disk behaves similarly to a solid one, with reductions in the force coefficients scaling with the cube of the pore radius. We also discover, perhaps surprisingly, that, as inertial effects intensify, the damping coefficient initially increases with the pore radius, reaches a maximum and subsequently declines as the disk’s inner hole enlarges further; at its peak, it can exceed the value for an equivalent solid disk by up to approximately 62% in the asymptotic limit of extremely high oscillatory Reynolds number. Conversely, the added mass coefficient decreases monotonically with increasing porosity. The decay rate of the added mass in the inertial regime initially scales with the cube of the pore radius before transitioning to linear scaling when the pore radius is no longer extremely small. Although our analysis assumes a small pore radius, direct numerical simulations confirm that our asymptotic formulation remains accurate for inner-to-outer radius ratios up to at least 1/2.
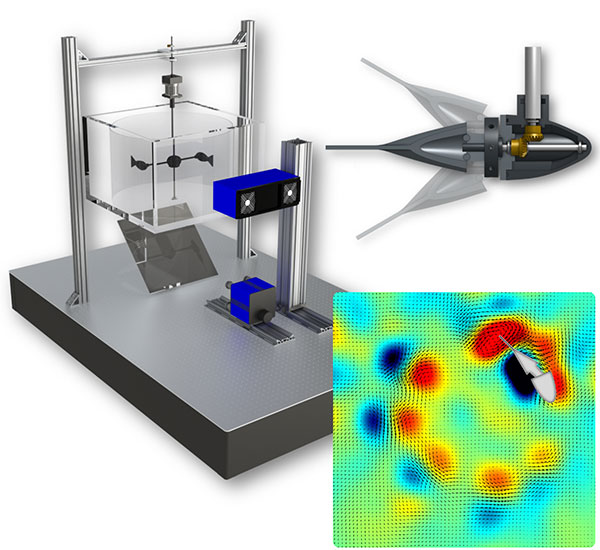
41. Multi-body hydrodynamic interactions in fish-like swimming
M. L. Timm, R. S. Pandhare, and H. Masoud, Applied Mechanics Reviews 76, 030801 (2024) pdf
Many animals in nature travel in groups either for protection, survival, or endurance. Among these, certain species do so under the burden of aero/hydrodynamic loads, which incites questions as to the significance of the multi-body fluid-mediated interactions that are inherent to collective flying/swimming. Prime examples of such creatures are fish, which are commonly seen traveling in highly organized groups of large numbers. Indeed, over the years, there have been numerous attempts to examine hydrodynamic interactions among self-propelled fish-like swimmers. Though many have studied this phenomenon, their motivations have varied from understanding animal behavior to extracting universal fluid dynamical principles and transplanting them into engineering applications. The approaches utilized to carry out these investigations include theoretical and computational analyses, field observations, and experiments using various abstractions of biological fish. Here, we compile representative investigations focused on the collective hydrodynamics of fish-like swimmers. The selected body of works are reviewed in the context of their methodologies and findings so as to draw parallels, contrast differences, and highlight open questions. Overall, the results of the surveyed studies provide foundational insights into the conditions (such as the relative positioning and synchronization between the members, as well as their swimming kinematics and speed) under which hydrodynamic interactions can lead to efficiency gains and/or group cohesion in two- and three-dimensional scenarios. They also shed some light on the mechanisms responsible for such energetic and stability enhancements in the context of wake-body, wake-wake, and body-body interactions.
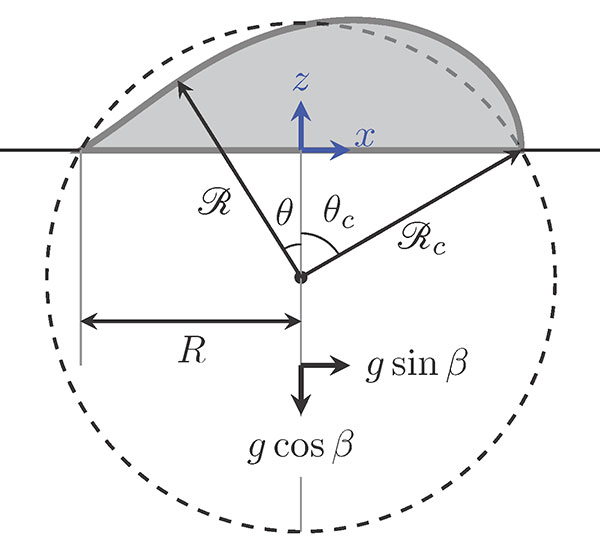
40. A second-order-accurate approximation for the shape of a sessile droplet deformed by gravity
M. L. Timm, R. S. M. Alassar, and H. Masoud, Journal of Engineering Mathematics 142, 5 (2023) pdf
We analytically solve the Young-Laplace equation for the shape of a stationary sessile droplet pinned to an inclined substrate, assuming that the droplet's contact line is circular. In the absence of gravity (or an equivalent external field), a sessile droplet takes the form of a spherical cap. Here, we calculate deviations from this ideal geometry when gravitational effects are non-negligible. Our calculations are based on a perturbation solution in powers of the Bond number Bo, which is a dimensionless parameter measuring the strength of gravity relative to surface tension. The newly derived solution is second-order accurate and builds on our previous work [Evaporation of a sessile droplet on a slope, Sci. Rep. 9, 19803 (2019)], where only the leading-order contributions were calculated. We consider the full range of substrate inclination angle from 0 to π and show that, when the second-order corrections are taken into account, the droplet's profile is captured more precisely, and the volume-conservation error of the solution is reduced considerably, all at a modest computational cost. We also find that our solution accurately approximates the gravity-induced deformation of the droplet for a wide range of droplet volumes and Bond numbers. As an example, we can very well predict the distorted shape of a droplet that is hemispherical at zero gravity up to Bo ≈ 4, 1.25, and 2.5 when the substrate is tilted from horizontal by 0, π/2, and π, respectively. Among other applications, the outcome of our study can serve as the first step toward analyzing the evaporation of sessile droplets deformed by gravity.
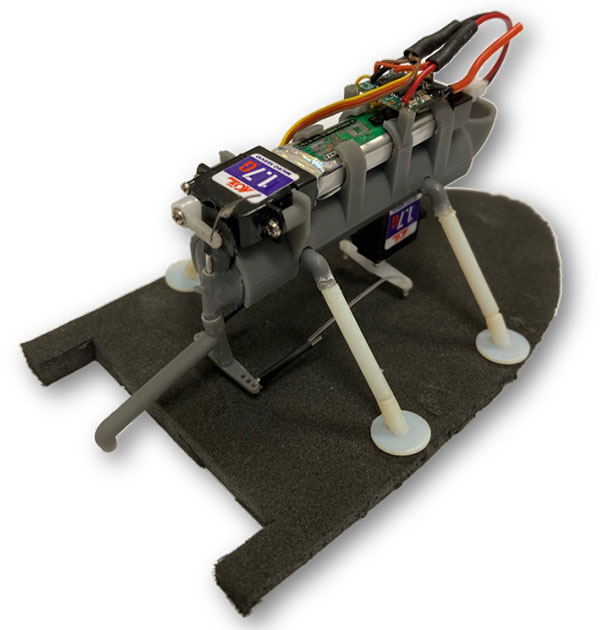
39. Using footpad sculpturing to enhance the maneuverability and speed of a robotic Marangoni surfer
S. Bechard, M. L. Timm, H. Masoud, and J. P. Rothstein, Biomimetics 8, 440 (2023) pdf
From insects to arachnids to bacteria, the surfaces of lakes and ponds are teaming with life. Many modes of locomotion are employed by these organisms to navigate along the air-water interface, including the use of lipid-laden excretions that can locally change the surface tension of the water and induce a Marangoni flow. In this paper, we improved the speed and maneuverability of a miniature remote-controlled robot that mimics insect locomotion using an onboard tank of isopropyl alcohol and a series of servomotors to control both the rate and location of alcohol release to both propel and steer the robot across the water. Here, we studied the effect of a series of design changes to the foam rubber footpads, which float the robot and are integral in efficiently converting the alcohol-induced surface tension gradients into propulsive forces and effective maneuvering. Two designs were studied: a two-footpad design and a single-footpad design. In the case of two footpads, the gap between the two footpads was varied to investigate its impact on straight-line speed, propulsion efficiency, and maneuverability. An optimal design was found with a small but finite gap between the two pads of 7.5 mm. In the second design, a single footpad without a central gap was studied. This footpad had a rectangular cut-out in the rear to capture the alcohol. Footpads with wider and shallower cut-outs were found to optimize efficiency. This observation was reinforced by the predictions of a simple theoretical mechanical model. Overall, the optimized single-footpad robot outperformed the two-footpad robot, producing a 30% improvement in speed and a 400% improvement in maneuverability.
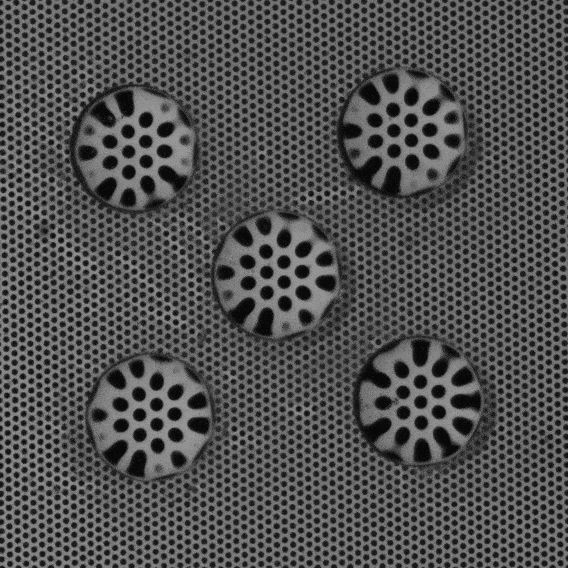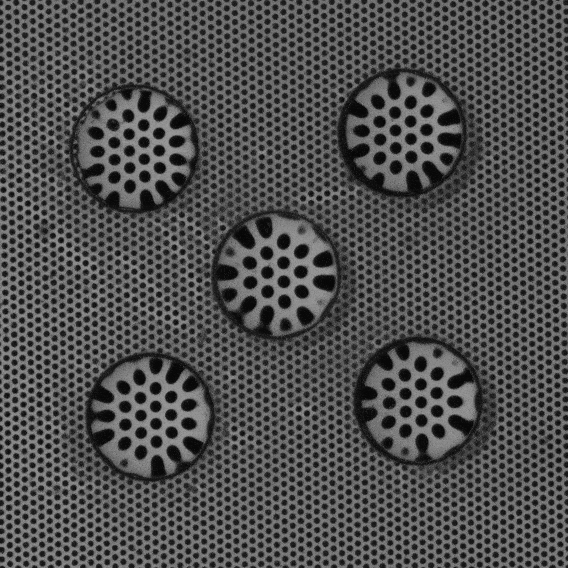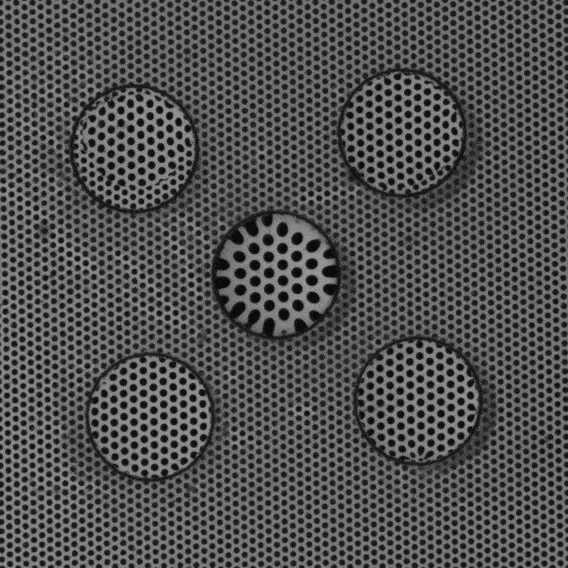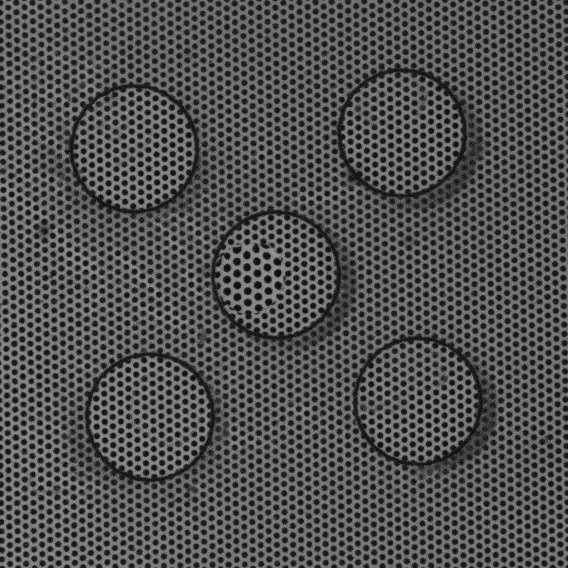
38. Drying dynamics of sessile-droplet arrays
A. Iqtidar, J. J. Kilbride, F. F. Ouali, D. J. Fairhurst, H. A. Stone, and H. Masoud, Physical Review Fluids 8, 013602 (2023) pdf
We analyze the diffusion-controlled evaporation of multiple droplets placed near each other on a planar substrate. Specifically, we calculate the change in the volume of sessile droplets with various initial contact angles that are arranged in different configurations. The calculations are supplemented by experimental measurements using a technique that interprets the variable magnification of a pattern placed beneath the droplet array, which is applied to the case of initially hemispherical droplets deposited in four distinct arrangements. We find excellent agreement between the predictions based on the theory of Masoud et al. [Evaporation of multiple droplets, J. Fluid Mech. 927, R4 (2021)] and the data gathered experimentally. Perhaps unexpectedly, we also find that, when comparing different arrays, the droplets with the same order of disappearance within their respective array, i.e., fastest evaporating, second-fastest evaporating, etc., follow similar drying dynamics. Our study provides not only experimental validation of the theoretical framework introduced by Masoud et al. but also offers additional insights into the evolution of the volume of individual droplets when evaporating within closely spaced arrays.
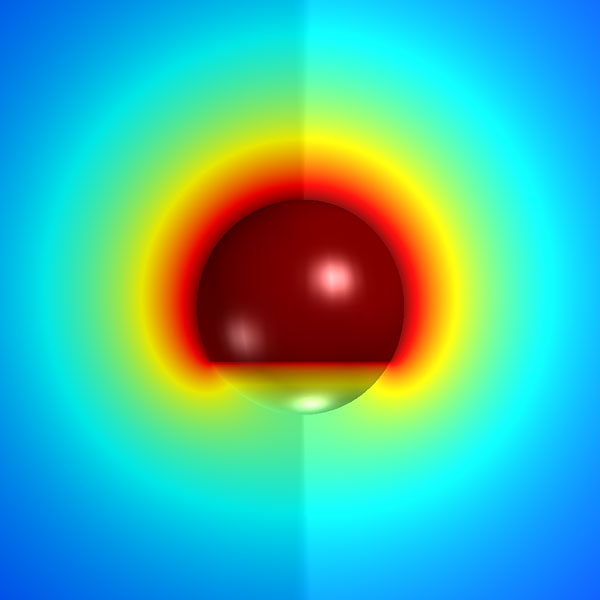
37. Diffusive mass transfer from a Janus sphere
H. Masoud and J. P. Rothstein, Physical Review Fluids 7, 070501 (2022) pdf
(Invited article for a special collection on Interfacial Active Matter)
We study the mixed boundary-value problem of finding the concentration distribution surrounding a two-sided (Janus) sphere, with a uniform concentration imposed on one segment of it and the no-mass-flux condition enforced on the other one. This mass transfer problem arises in the analysis of the Marangoni propulsion phenomenon, where a chemically active particle propels itself along a liquid-gas interface via nonuniform release of a chemical species that locally alters the surface tension distribution. Assuming that the mass transfer is purely driven by diffusion (i.e., neglecting advection), we present a novel approximate form for the concentration field derived from the integral representation of the solution to the corresponding Laplace equation. We demonstrate the high fidelity of the proposed approach by comparing its results with those obtained from a collocation method based on the series solution of the problem. Beyond the motivating problem, our findings are expected to be applicable to the self-diffusiophoresis of catalytic colloids, as well as to the conduction heat transfer and electrostatics problems involving partially insulated spheres. Moreover, our approach can lead to the derivation of similarly accurate approximate solutions to analogous problems in mathematical physics.
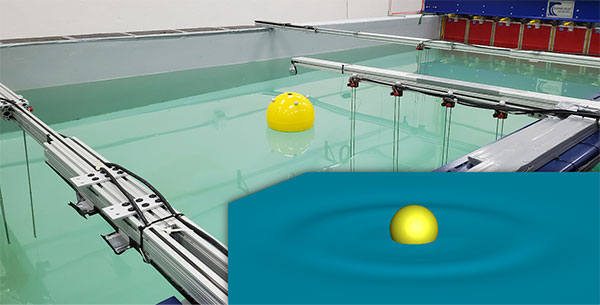
36. Free-decay heave motion of a spherical buoy
J. K. Colling, S. Jafari Kang, E. Dehdashti, S. Husain, H. Masoud, and G. G. Parker, Fluids 7, 188 (2022) pdf
(Invited article for a special issue on Fluid-Structure Interaction: Methods and Applications)
(Selected by editors as cover story)
We examined the heave motion of a spherical buoy during a free-decay drop test. A comprehensive approach was adopted to study the oscillations of the buoy involving experimental measurements and complementary numerical simulations. The experiments were performed in a wave tank equipped with an array of high-speed motion-capture cameras and a set of high-precision wave gauges. The simulations included three sets of calculations with varying levels of sophistication. Specifically, in one set, the volume-of-fluid (VOF) method was used to solve the incompressible, two-phase, Navier–Stokes equations on an overset grid, whereas the calculations in other sets were based on Cummins and mass-spring-damper models that are both rooted in the linear potential flow theory. Excellent agreements were observed between the experimental data and the results of VOF simulations. Although less accurate, the predictions of the two reduced-order models were found to be quite credible, too. Regarding the motion of the buoy, the obtained results indicate that, after being released from a height approximately equal to its draft at static equilibrium (which is about 60% of its radius), the buoy underwent nearly harmonic damped oscillations. The analysis that was conducted reveals that the draft length of the buoy has a profound effect on the frequency and attenuation rate of the oscillations. For example, compared to a spherical buoy of the same size that is half-submerged at equilibrium (i.e., the draft is equal to the radius), the tested buoy oscillated with a period that was roughly 20% shorter, and its amplitude of oscillations decayed almost twice faster per period. Overall, the presented study provides additional insights into the motion response of a floating sphere that can be used for optimal buoy design for energy extraction.
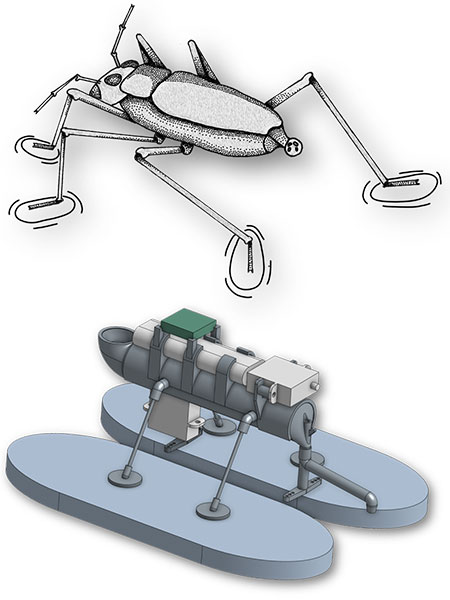
35. A remotely controlled Marangoni surfer
M. L. Timm, S. Jafari Kang, J. P. Rothstein, and H. Masoud, Bioinspiration & Biomimetics 16, 066014 (2021) pdf
(Highlighted in Michigan Tech's Unscripted Research Blog, Tech Explore, and Nanowerk)
Inspired by creatures that have naturally mastered locomotion on the air-water interface, we developed and built a self-powered, remotely controlled surfing robot capable of traversing this boundary by harnessing surface tension modification for both propulsion and steering through a controlled release of isopropyl alcohol. In this process, we devised and implemented novel release valve and steering mechanisms, culminating in a surfer with distinct capabilities. Our robot measures about 110 mm in length and can travel as fast as 0.8 body length per second. Interestingly, we found that the linear speed of the robot follows a 1/3 power law with the release rate of the propellant. Additional maneuverability tests also revealed that the robot is able to withstand 20 mm/s² in centripetal acceleration while turning. Here, we thoroughly discuss the design, development, performance, overall capabilities, and ultimate limitations of our robotic surfer.
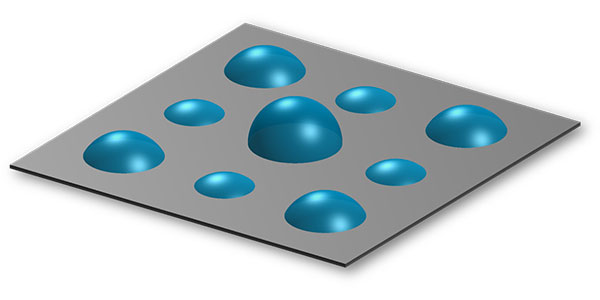
34. Evaporation of multiple droplets
H. Masoud, P. D. Howell, and H. A. Stone, Journal of Fluid Mechanics (Rapids) 927, R4 (2021) pdf
(Highlighted in a Focus on Fluids article by JFM)
We derive an accurate estimate for the diffusive evaporation rates of multiple droplets of different sizes and arbitrary contact angles placed on a horizontal substrate. The derivation, which is based on a combination of Green's second identity and the method of reflections, simply makes use of the solution for the evaporation of a single droplet. The theoretical results can serve as a guide for future computational and experimental studies on the collective evaporation of arrays of droplets, as well as similar multi-body, diffusion-dominated transport problems.
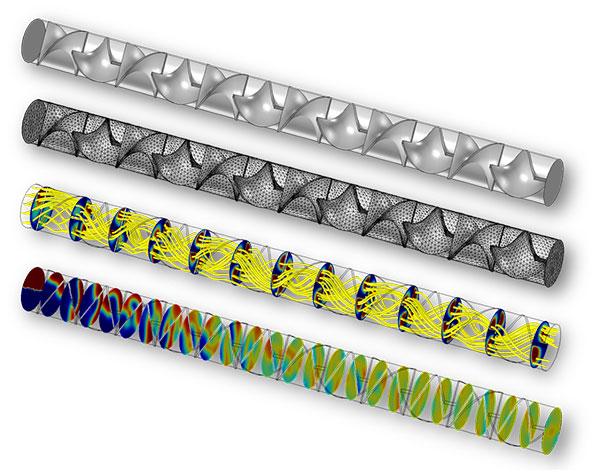
33. Continuous purification of an enveloped and non-enveloped viral particle using an aqueous two-phase system
D. G. Turpeinen, P. U. Joshi, S. A. Kriz, S. Kaur, N. M. Nold, D. O'Hagan, S. Nikam, H. Masoud, and C. L. Heldt, Separation and Purification Technology 269, 118753 (2021) pdf
Meeting the increasing demand for vaccines throughout the world is key to decreasing the spread of infectious diseases. The switch to a fully continuous vaccine manufacturing process would increase productivity and the supply of crucial vaccines. To aid in this switch, we have developed a novel, continuous downstream purification technique based on an aqueous two-phase system (ATPS). The system has the potential to be used as a platform system for viral product purification. A 12 kDa poly(ethylene glycol) (PEG) and trisodium citrate ATPS were able to purify porcine parvovirus (PPV) and human immunodeficiency virus type-1 group antigen virus-like particles (HIV VLPs) from the cell supernatant. PPV was recovered in the PEG-rich phase at 90 ± 16% with a DNA removal of 96 ± 3% and ≥ 89 protein removal. The system was also able to recover 99 ± 2% of HIV VLPs in the PEG-rich phase with a 73 ± 1% DNA removal and high protein removal shown by SDS-PAGE. Continuous ATPS recovered the virus at the same amount as batch recovery. This demonstrates that continuous ATPS can be scaled up and run continuously without a loss in purity or recovery. Mixing and settling time both played an important role in developing a continuous ATPS for viral particles.
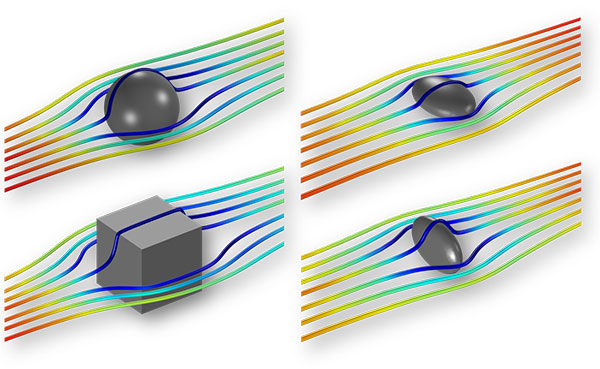
32. Heat transfer from a particle in laminar flows of a variable thermal conductivity fluid
E. Dehdashti, M. Razizadeh, and H. Masoud, International Journal of Heat and Mass Transfer 171, 121067 (2021) pdf
We revisit the classical problem of steady-state heat transfer from a single particle in a uniform laminar flow with the assumption that the thermal conductivity of the fluid changes linearly with the temperature. We use a combination of asymptotic and scaling analyses to derive approximate expressions for the dimensionless heat transfer coefficient, i.e., the Nusselt number Nu, of arbitrarily shaped particles. The results cover the entire range of the Peclet number Pe. We find that, for a constant temperature boundary condition and fixed geometry, the Nusselt number is essentially equal to the product of two terms, one of which is only a function of Pe, while the other one is nearly independent of Pe and mainly depends on the proportionality constant of the conductivity-temperature relation. We also show that, in contrast, when a uniform heat flux is imposed on the surface of the particle, Nu can be estimated as a summation of a Pe-dependent piece and one that solely varies with the proportionality constant.
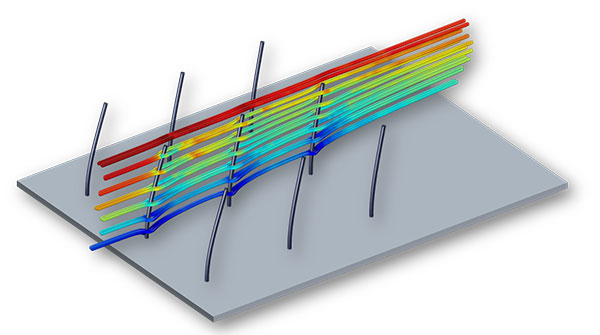
31. Collective sensitivity of artificial hair sensors to flow direction
E. Dehdashti, G. W. Reich, and H. Masoud, AIAA Journal 59, 1135-1141 (2021) pdf
We study the collective response of synthetic hair sensors to changes in the direction of airflow. Specifically, we examine whether an array of isotropic sensors – that are unable to detect the direction of an incident flow when operating in isolation – can together sense not only the magnitude but also the orientation of a free-stream flow passing over and through them. To conduct our inquiry, we consider a fundamental scenario involving a boundary-layer flow past a flat plate decorated with a 3 by 3 array of sensors. Numerical modeling is used to simulate the interaction between the fluid flow and flexible hair sensors. We find that, indeed, it is feasible to achieve collective directional sensitivity by engineering the arrangement of the sensors. We also propose a supervised machine-learning approach capable of deducing the direction of the free-stream flow from the deformation signals outputted by individual sensors in the array.
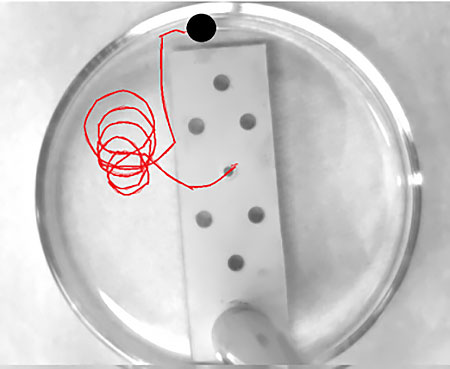
30. The Effect of Shape on the Motion and Stability of Marangoni Surfers
S. Sur, N. Uvanovic, H. Masoud, and J. P. Rothstein, Journal of Fluids Engineering 143, 011301 (2021) pdf
The Marangoni propulsion of spheres and elliptical disks floating on the air-water interface was studied to understand the effect of particle shape on its motion and its stability at moderate Reynolds numbers. Self-propulsion of the Marangoni surfer was achieved by coating half of the spheres and the elliptical disks with either a solution of soap or isopropyl alcohol. The presence of soap or isopropyl alcohol resulted in a gradient of surface tension across the particles, which propelled the particles in the direction of increasing surface tension. The velocity of the particles was increased by increasing the concentration of soap or isopropyl alcohol. Beyond a critical velocity, a transition was observed from a straight-line motion to a rotational motion. Using Particle Image Velocimetry, the flow field around the spheres and elliptical disks was measured in order to investigate the origin of their rotational motion. With increasing Reynolds number, interfacial vortices attached to the sides of the particles were observed to form and strengthen. These vortices were observed to shed above a critical Reynolds number, resulting in an unbalanced torque that caused the particles to rotate. Increasing the aspect ratio between the major and minor axes of the elliptical disks was found to decrease their stability and greatly enhance their rate of rotation. This was especially true for elliptical disks traveling in a direction parallel to their major axis. The interactions between the particles and the wall of a Petri dish were also studied. Due to its density and shape, the interface around the floating sphere was deformed, resulting in a convex interfacial curvature that interacted with the interfacial curvature imposed along the confining wall of the Petri dish. Repulsive, concave curvature was found to decrease stability and enhance rotational motion, while attractive, convex curvature was shown to stabilize the straight-line motion of the spheres. For the neutrally buoyant elliptical disks, the presence of the bounding wall was found to greatly stabilize the straight-line motion of the elliptical disks when they were traveling in a direction parallel to their minor axis.
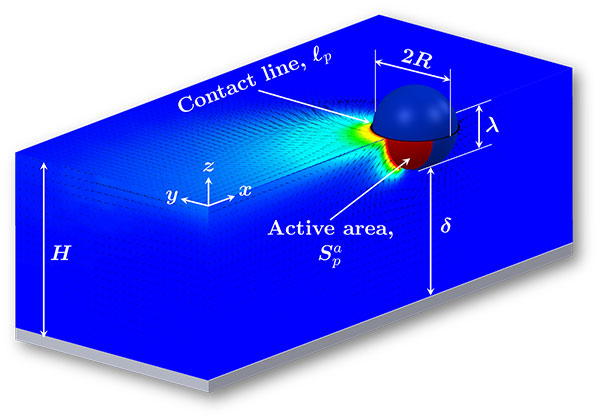
29. Forward, reverse, and no motion of Marangoni surfers under confinement
S. Jafari Kang, S. Sur, J. P. Rothstein, and H. Masoud, Physical Review Fluids 5, 084004 (2020) pdf
(Highlighted in Michigan Tech News, Phys.org, Science Daily, and Nanowerk)
We examine the mobility of a chemically active particle straddling the interface between a liquid layer of finite depth and a semi-infinite layer of gas. A surface-active agent is released asymmetrically from the particle that locally lowers the interfacial surface tension. It is commonly presumed that the uneven distribution of the surface tension and the associated Marangoni flow leads to the propulsion of the active surfer opposite the release direction, where the surface tension is higher. This is considered forward motion. However, our recent theoretical analysis – within the limits of negligible inertia and diffusion-dominated transport of the active agent – has shown that this trend may be reversed for certain shapes of the surfer and shallow enough liquid layers. Advancing beyond the Stokes regime, here, we study the Marangoni-driven motion of thin cylindrical disks and oblate spheroids for a wide range of release rates and diffusivity of the exuded chemical species that control the effective Reynolds and Peclet numbers. We consider various degrees of confinement represented by the thickness of the liquid film and show that, indeed, the surfers can undergo a forward, backward, or arrested motion. We also identify the links between these modes of mobility and the forces acting on the surfers, as well as the flow structure in their vicinity. Rather unexpectedly, we discover that negative pressure is the primary contributor to the fluid force experienced by the surfer and that this suction force is mainly responsible for the reverse Marangoni propulsion. The reported results are based on closely corroborating numerical calculations and experimental measurements.
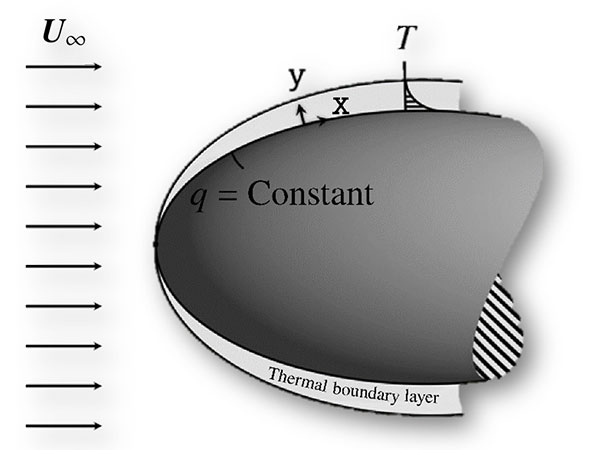
28. Forced convection heat transfer from a particle at small and large Peclet numbers
E. Dehdashti and H. Masoud, Journal of Heat Transfer 142, 061803 (2020) pdf
We theoretically study forced convection heat transfer from a single particle in uniform laminar flows. Asymptotic limits of small and large Peclet numbers Pe are considered. For Pe ≪ 1 (diffusion-dominated regime) and a constant heat flux boundary condition on the surface of the particle, we derive a closed-form expression for the heat transfer coefficient that is valid for arbitrary particle shapes and Reynolds numbers, as long as the flow is incompressible. Remarkably, our formula for the average Nusselt number Nu is identical to the one obtained by Brenner for a uniform temperature boundary condition (Chem. Eng. Sci., vol. 18, 1963, pp. 109--122). We also present a framework for calculating the average Nu of axisymmetric and two-dimensional objects with a constant heat flux surface condition in the limits of Pe ≫ 1 and small or moderate Reynolds numbers. Specific results are presented for the heat transfer from spheroidal particles in Stokes flow.

27. How to walk on water and climb up walls: Animal movement and the robots of the future
H. Masoud, American Journal of Physics 88, 423 (2020) pdf
(Invited book review)
It is hard not to be fascinated by a book written by a scientist who, in the last five years, won two Ig Nobel Prizes – for his works on the urination of mammals (which is covered in the book) and the shape of wombat poo. A parody of the Nobel Prize, the award honors research accomplishments that appear funny (or even useless) at first glance, whereas, upon a deeper look, one realizes that they reveal keen scientific discoveries. Indeed, what makes this book special is the distinct personality and research style of its author. David Hu is a true cross-disciplinary researcher. He is an engineer, a mathematician, a physicist, and a biologist, either by formal training or self-learning. By the time you have finished reading the book, you will agree with me (and the American Institute of Physics judges who selected David for the 2019 AIP Science Communication Award) that he is also a very effective communicator of science to non-specialists.
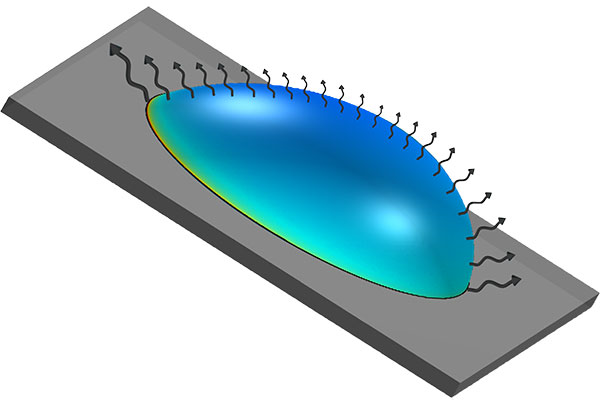
26. Evaporation of a sessile droplet on a slope
M. L. Timm, E. Dehdashti, A. Jarrahi Darban, and H. Masoud, Scientific Reports 9, 19803 (2019) pdf
We theoretically examine the drying of a stationary liquid droplet on an inclined surface. Both analytical and numerical approaches are considered while assuming that the evaporation results from the purely diffusive transport of liquid vapor and that the contact line is a pinned circle. For the purposes of the analytical calculations, we suppose that the effect of gravity relative to the surface tension is weak, i.e., the Bond number (Bo) is small. Then, we express the shape of the drop and the vapor concentration field as perturbation expansions in terms of Bo. When the Bond number is zero, the droplet is unperturbed by the effect of gravity and takes the form of a spherical cap, for which the vapor concentration field is already known. Here, the Young-Laplace equation is solved analytically to calculate the first-order correction to the shape of the drop. Knowing the first-order perturbation to the drop geometry and the zeroth-order distribution of vapor concentration, we obtain the leading-order contribution of gravity to the rate of droplet evaporation by utilizing Green's second identity. The analytical results are supplemented by numerical calculations, where the droplet shape is first determined by minimizing the Helmholtz free energy, and then the evaporation rate is computed by solving Laplace's equation for the vapor concentration field via a finite-volume method. Perhaps counter-intuitively, we find that even when the droplet deforms noticeably under the influence of gravity, the rate of evaporation remains almost unchanged, as if no gravitational effect is present. Furthermore, a comparison between analytical and numerical calculations reveals that considering only the leading-order corrections to the shape of the droplet and vapor concentration distribution provides estimates that are valid well beyond their intended limit of very small Bo.
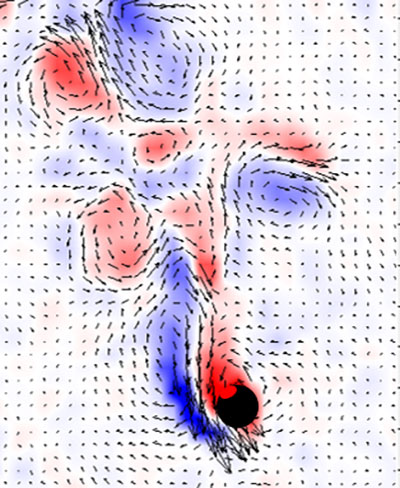
25. Translational and rotational motion of disk-shaped Marangoni surfers
S. Sur, H. Masoud, and J. P. Rothstein, Physics of Fluids 31, 102101 (2019) pdf
In this paper, we study the Marangoni propulsion of a neutrally buoyant disk-shaped object at the air-water interface. Self-propulsion was achieved by coating the back of the disk with either soap or isopropyl alcohol in order to generate and then maintain a surface tension gradient across the surfer. As the propulsion strength and the resulting disk velocity were increased, a transition from a straight-line translational motion to a rotational motion was observed. Although spinning had been observed before for asymmetric objects, these are the first observations of spinning of a geometrically axisymmetric Marangoni surfer. Particle tracking and particle image velocimetry measurements were used to interrogate the resulting flow field and understand the origin of the rotational motion of the disk. These measurements showed that as the Reynolds number was increased, interfacial vortices attached to the sides of the disk were formed and intensified. Beyond a critical Reynolds number of Re > 120, a vortex was observed to shed, resulting in an unbalanced torque on the disk that caused it to rotate. The interaction between the disk and the confining wall of the Petri dish was also studied. Upon approaching the bounding wall, a transition from straight-line motion to rotational motion was observed at significantly lower Reynolds numbers than on an unconfined interface. Interfacial curvature was found to either enhance or eliminate rotational motion depending on whether the curvature was repulsive (concave) or attractive (convex).
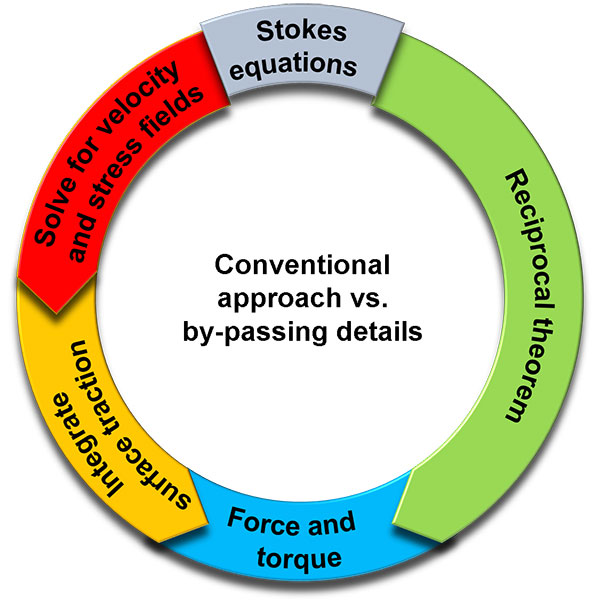
24. The reciprocal theorem in fluid dynamics and transport phenomena
H. Masoud and H. A. Stone, Journal of Fluid Mechanics 879, P1 (2019) pdf
(Invited "Perspectives" article)
In the study of fluid dynamics and transport phenomena, key quantities of interest are often the force and torque on objects and the total rate of heat/mass transfer from them. Conventionally, these integrated quantities are determined by first solving the governing equations for the detailed distribution of the field variables (i.e., velocity, pressure, temperature, concentration, etc.) and then integrating the variables or their derivatives on the surface of the objects. On the other hand, the divergence form of the conservation equations opens the door for establishing integral identities that can be used for directly calculating the integrated quantities without requiring detailed knowledge of the distribution of the primary variables. This shortcut approach constitutes the idea of the reciprocal theorem, whose closest relative is Green’s second identity, which readers may recall from studies of partial differential equations. Despite its importance and practicality, the theorem may not be so familiar to many in the research community. Ironically, some believe that the extreme simplicity and generality of the theorem are responsible for suppressing its application! In this Perspectives piece, we provide a pedagogical introduction to the concept and application of the reciprocal theorem with the hope of facilitating its use. Specifically, a brief history of the development of the theorem is given as a background, followed by a discussion of the main ideas in the context of elementary boundary-value problems. After that, we demonstrate how the reciprocal theorem can be utilized to solve fundamental problems in low-Reynolds-number hydrodynamics, aerodynamics, acoustics and heat/mass transfer, including convection. Throughout the article, we strive to make the materials accessible to early career researchers while keeping it interesting for more experienced scientists and engineers.
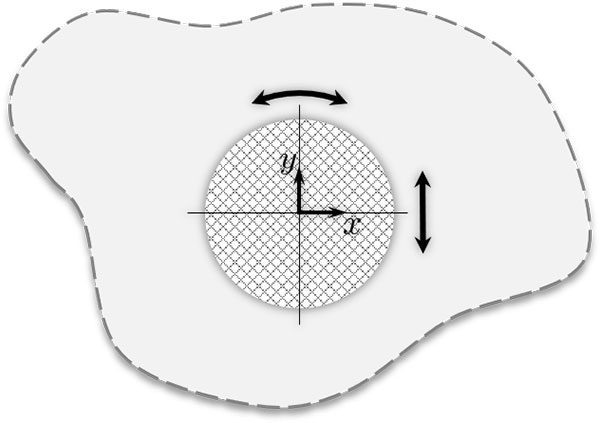
23. Optimal viscous damping of vibrating porous cylinders
S. Jafari Kang, E. Dehdashti, V. Vandadi, and H. Masoud, Journal of Fluid Mechanics 874, 339-358 (2019) pdf
We theoretically study small-amplitude oscillations of permeable cylinders immersed in an unbounded fluid. Specifically, we examine the effects of oscillation frequency, permeability, and shape on the effective mass and damping coefficients, the latter of which is proportional to the power required to sustain the vibrations. Cylinders of circular and elliptical cross-sections undergoing transverse and rotational vibrations are considered. The dynamics of the fluid flow through porous cylinders is assumed to obey the unsteady Brinkman-Debye-Bueche equations. We use a singularity method to analytically calculate the flow field within and around circular cylinders, whereas we introduce a Fourier-pseudospectral method to numerically solve the governing equations for elliptical cylinders. We find that, if rescaled properly, the analytical results for circular cylinders provide very good estimates for the behavior of elliptical ones over a wide range of conditions. More importantly, our calculations indicate that, at sufficiently high frequencies, the damping coefficient of oscillations varies non-monotonically with the permeability, in which case it maximizes when the diffusion length scale for the vorticity is comparable to the penetration length scale for the flow within the porous material. Depending on the oscillation period, the maximum damping of a permeable cylinder can be many times greater than that of an otherwise impermeable one. This might seem counter-intuitive at first since, generally, the power it takes to steadily drag a permeable object through a fluid is less than the power needed to drive the steady motion of the same impermeable object. However, the driving power (or damping coefficient) for oscillating bodies is determined by not only the amplitude of the cyclic fluid load experienced by them but also by the phase shift between the load and their periodic motion. An increase in the latter is responsible for the excess damping coefficient of vibrating porous cylinders.
22. Conduction heat transfer from oblate spheroids and bispheres
S. Jafari Kang, E. Dehdashti, and H. Masoud, International Journal of Heat and Mass Transfer 139, 115-120 (2019) pdf
We theoretically examine heat transfer by conduction from oblate spheroidal and bispherical surfaces into a stationary, infinite medium. The surfaces are presumed to maintain a constant heat flux. Assuming steady-state conditions and uniform thermal conductivity, we analytically solve Laplace’s equation for the temperature distribution and discuss the challenge of dealing with the Neumann (uniform flux) versus the more convenient Dirichlet (isothermal) boundary condition. The solutions are obtained in boundary-fitting coordinate systems using the method of separation of variables and eigenfunction expansion. The exact expressions for the average Nusselt number are presented along with their approximations.
21. Fluid flows with interactive boundaries
H. Masoud and A. M. Ardekani, European Journal of Computational Mechanics 26, 1-3 (2017) pdf
(Editorial article for a special issue)
One of the most fascinating and non-intuitive class of problems in fluid mechanics are those involving the interplay between dynamic boundaries and fluid flows. The coupling between the dynamics of the fluid and the kinematics and mechanics of the boundaries in these situations often gives rise to unexpected behaviors that are of paramount importance in many engineering, biological and biomedical contexts. The study of problems involving fluid flows with interactive boundaries has been the focus of the field in the past two decades, to the extent that many examples of such problems have frequently appeared on the cover of recent editions of fluid mechanics journals and textbooks. This relatively new and exciting frontier in fluid mechanics is also highly interdisciplinary and has attracted many engineers and scientists alike.
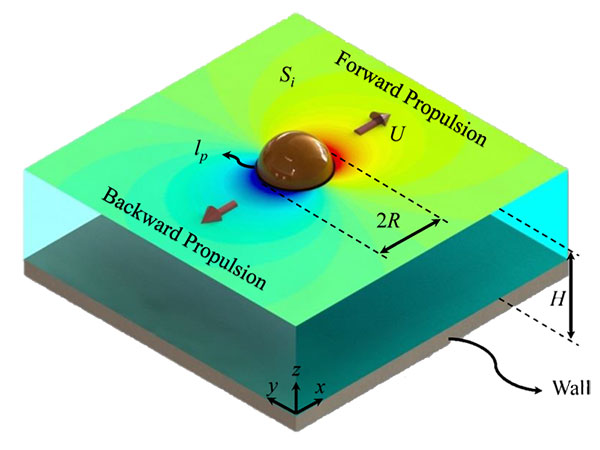
20. Reverse Marangoni surfing
V. Vandadi, S. Jafari Kang, and H. Masoud, Journal of Fluid Mechanics 811, 612-621 (2017) pdf
We theoretically study the surfing motion of chemically and thermally active particles located at a flat liquid-gas interface that sits above a liquid layer of finite depth. The particles' activity creates and maintains a surface tension gradient, resulting in auto-surfing. It is intuitively perceived that Marangoni surfers propel towards the direction with a higher surface tension. Remarkably, we find that the surfers may propel in the lower surface tension direction depending on their geometry and proximity to the bottom of the liquid layer. In particular, our analytical calculations for Stokes flow and diffusion-dominated scalar fields (i.e., chemical concentration and temperature fields) indicate that spherical particles undergo reverse Marangoni propulsion under confinement, whereas disk-shaped surfers always move in the expected direction. We extend our results by proposing an approximate formula for the propulsion speed of oblate spheroidal particles based on the speeds of spheres and disks.
19. Alternative mechanism for coffee-ring deposition based on active role of free surface
S. Jafari Kang, V. Vandadi, J. D. Felske, and H. Masoud, Physical Review E 94, 063104 (2016) pdf
(Highlighted in Materials Today, Nevada Today, Phys.org, Science Daily, Membrane Quarterly, and Nanowerk)
When a colloidal sessile droplet dries on a substrate, the particles suspended in it usually deposit in a ring-like pattern. This phenomenon is commonly referred to as the "coffee-ring" effect. One paradigm for why this occurs is as a consequence of the solutes being transported towards the pinned contact line by the flow inside the drop, which is induced by surface evaporation. From this perspective, the role of the liquid-gas interface in shaping the deposition pattern is somewhat minimized. Here, we propose an alternative mechanism for the coffee-ring deposition. It is based on the bulk flow within the drop transporting particles to the interface, where they are captured by the receding free surface and subsequently transported along the interface until they are deposited near the contact line. That the interface captures the solutes as the evaporation proceeds is supported by a Lagrangian tracing of particles advected by the flow field within the droplet. We model the interfacial adsorption and transport of particles as a one-dimensional advection-generation process in toroidal coordinates and show that the theory reproduces ring-shaped depositions. Using this model, deposition patterns on both hydrophilic and hydrophobic surfaces are examined, in which the evaporation is modelled as being either diffusive or uniform over the surface.
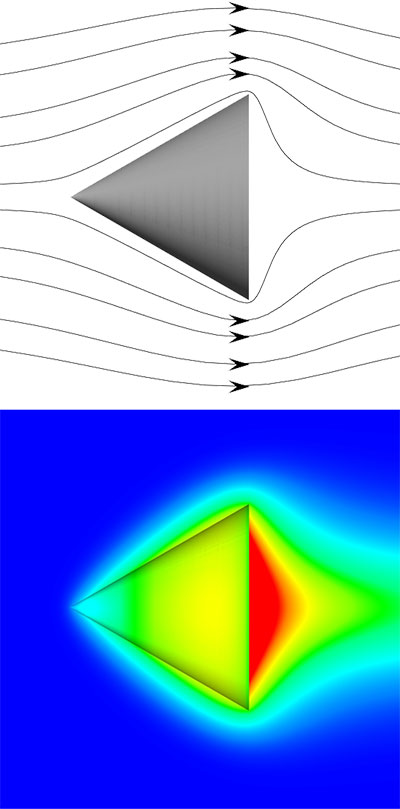
18. Reciprocal theorem for convective heat and mass transfer from a particle in Stokes and potential flows
V. Vandadi, S. Jafari Kang, and H. Masoud, Physical Review Fluids (Rapid Communications) 1, 022001(R) (2016) pdf
In the study of convective heat and mass transfer from a particle, key quantities of interest are usually the average rate of transfer and mean distribution of the scalar (i.e., temperature or concentration) at the particle surface. Calculating these quantities using conventional equations requires detailed knowledge of the scalar field, which is available predominantly for problems involving uniform scalar and flux boundary conditions. Here, we derive a reciprocal relation between two diffusing scalars that are advected by oppositely driven Stokes or potential flows whose streamline configurations are identical. This relation leads to alternative expressions for the aforementioned average quantities based on the solution of the scalar field for uniform surface conditions. We exemplify our results via two applications: (i) heat transfer from a sphere with non-uniform boundary conditions in Stokes flow at small Peclet numbers, and (ii) extension of Brenner's theorem for the invariance of heat transfer rate to flow reversal.
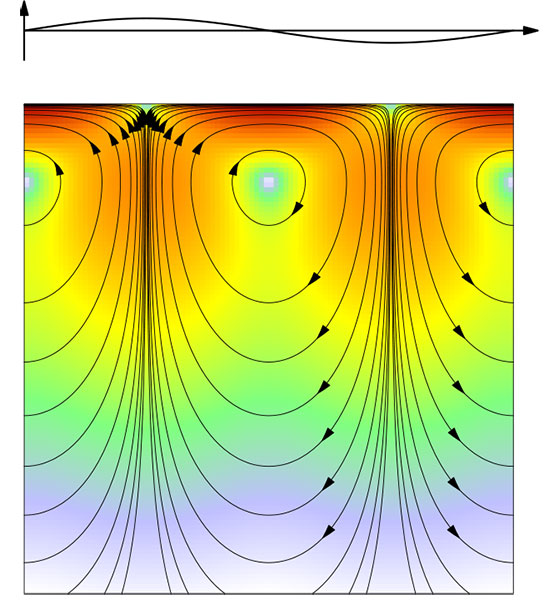
17. Oscillatory Marangoni flows with inertia
O. Shardt, H. Masoud, and H. A. Stone, Journal of Fluid Mechanics 803, 94-118 (2016) pdf
When the surface of a liquid has a non-uniform distribution of a surfactant that lowers surface tension, the resulting variation in surface tension drives a flow that spreads the surfactant towards a uniform distribution. We study the spreading dynamics of an insoluble and non-diffusing surfactant on an initially motionless liquid. We derive solutions for the evolution over time of sinusoidal variations in surfactant concentration with a small initial amplitude relative to the average concentration. In this limit, the coupled flow and surfactant transport equations are linear. In contrast to exponential decay when the inertia of the flow is negligible, the solution for unsteady Stokes flow exhibits oscillations when inertia is sufficient to spread the surfactant beyond a uniform distribution. This oscillatory behavior exhibits two properties that distinguish it from that of a simple harmonic oscillator: the amplitude changes sign at most three times, and the decay at late times follows a power law with an exponent of −3/2. As the surface oscillates, the structure of the subsurface flow alternates between one and two rows of counter-rotating vortices, starting with one row and ending with two during the late-time monotonic decay. We also examine numerically the evolution of the surfactant distribution when the system is nonlinear due to a large initial amplitude.
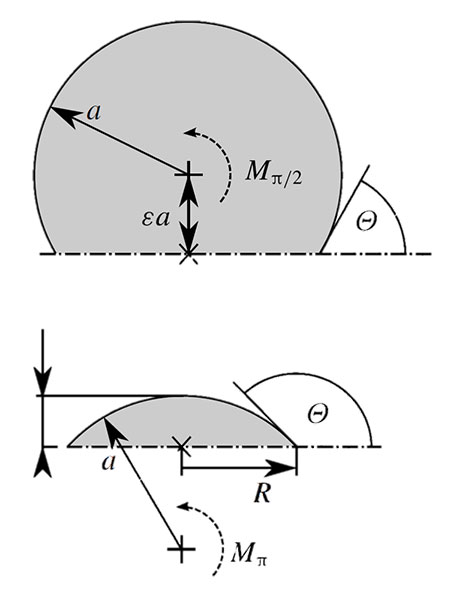
16. Drag and diffusion coefficients of a spherical particle attached to a fluid-fluid interface
A. Dörr, S. Hardt, H. Masoud, and H. A. Stone, Journal of Fluid Mechanics 790, 607-618 (2016) pdf
Explicit analytical expressions for the drag and diffusion coefficients of a spherical particle attached to the flat interface between two immiscible fluids are constructed for the case of a vanishing viscosity ratio between the fluid phases. The model is designed to account explicitly for the dependence on the contact angle between the two fluids and the solid surface. The Lorentz reciprocal theorem is applied in the context of geometric perturbations from the limiting cases of 90o and 180o contact angles. The model agrees well with the experimental and numerical data from the literature. Also, an advantage of the method utilized is that the drag and diffusion coefficients can be calculated up to one order higher in the perturbation parameter than the known velocity and pressure fields. Extensions to other particle shapes with known velocity and pressure fields are straightforward.
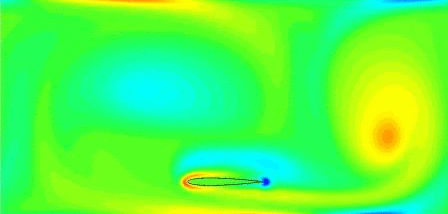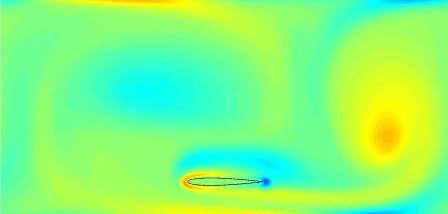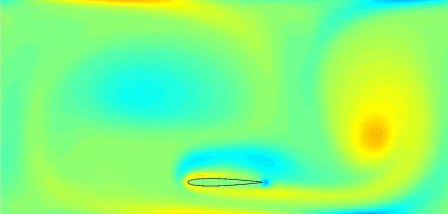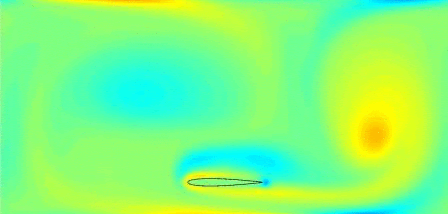
15. Hydrodynamic schooling of flapping swimmers
A. Becker*, H. Masoud*, J. Newbolt, M. J. Shelley, and L. Ristroph, Nature Communications 6, 8514 (2015) pdf
(*Authors of equal contribution)
(Highlighted in APS Physics Central Podcast, Science Daily, and Futurity)
Fish schools and bird flocks are fascinating examples of collective behaviors in which many individuals generate and interact with complex flows. Motivated by animal groups on the move, here we explore how the locomotion of many bodies emerges from their flow-mediated interactions. Through experiments and simulations of arrays of flapping wings that propel within a collective wake, we discover distinct modes characterized by the group swimming speed and the spatial phase shift between trajectories of neighboring wings. For identical flapping motions, slow and fast modes coexist and correspond to constructive and destructive wing–wake interactions. Simulations show that swimming in a group can enhance speed and save power, and we capture the key phenomena in a mathematical model based on memory or the storage and recollection of information in the flow field. These results also show that fluid dynamic interactions alone are sufficient to generate coherent collective locomotion and thus might suggest new ways to characterize the role of flows in animal groups.
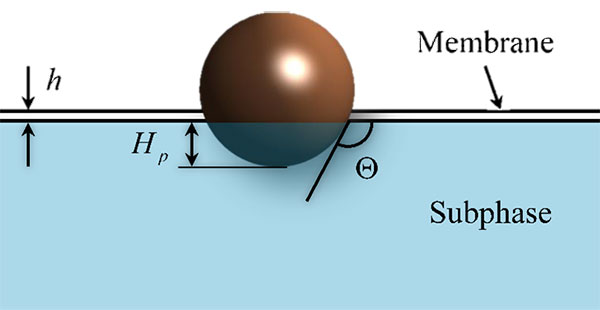
14. Mobility of membrane-trapped particles
H. A. Stone and H. Masoud, Journal of Fluid Mechanics 781, 494-505 (2015) pdf
Rheological and transport studies of model thin films and membranes, often inspired by biological systems, make use of translational or rotational motion or diffusion of particles trapped in the surface film. Here, we consider the translational mobility of spherical and oblate spheroidal particles protruding into the surrounding subphase liquid. Both the subphase and surface film contribute to the resistance experienced by the particle, which is calculated as a function of the degree of protrusion as well as the viscosity contrast between the surface film and the surrounding fluid. The calculations are based on a combination of a perturbation expansion involving the particle shape and the Lorentz reciprocal theorem.
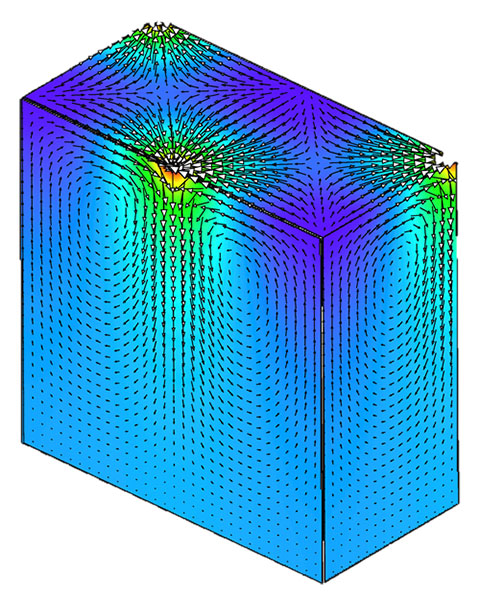
13. Collective surfing of chemically active particles
H. Masoud and M. J. Shelley, Physical Review Letters 112, 128304 (2014) pdf
(Highlighted as PRL Editors' Suggestion)
We study theoretically the collective dynamics of immotile particles bound to a 2D surface atop a 3D fluid layer. These particles are chemically active and produce a chemical concentration field that creates surface-tension gradients along the surface. The resultant Marangoni stresses create flows that carry the particles, possibly concentrating them. For a 3D diffusion-dominated concentration field and Stokesian fluid, we show that the surface dynamics of active particle density can be determined using nonlocal 2D surface operators. Remarkably, we also show that for both deep and shallow fluid layers, this surface dynamics reduce to the 2D Keller-Segel model for the collective chemotactic aggregation of slime mold colonies. Mathematical analysis has established that the Keller-Segel model can yield finite-time, finite-mass concentration singularities. We show that such singular behavior occurs in our finite-depth system and study the associated 3D flow structures.
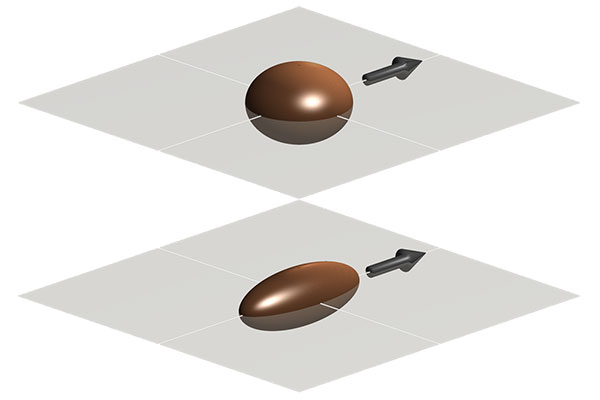
12. A reciprocal theorem for Marangoni propulsion
H. Masoud and H. A. Stone, Journal of Fluid Mechanics (Rapids) 741, R4 (2014) pdf
We study the Marangoni propulsion of a spheroidal particle located at a liquid-gas interface. The particle asymmetrically releases an insoluble surface-active agent and so creates and maintains a surface tension gradient, leading to self-propulsion. Assuming that the surface tension has a linear dependence on the concentration of the released agent, we derive closed-form expressions for the translational speed of the particle in the limit of small capillary, Péclet and Reynolds numbers. Our derivations are based on the Lorentz reciprocal theorem, which eliminates the need to develop a detailed flow field.
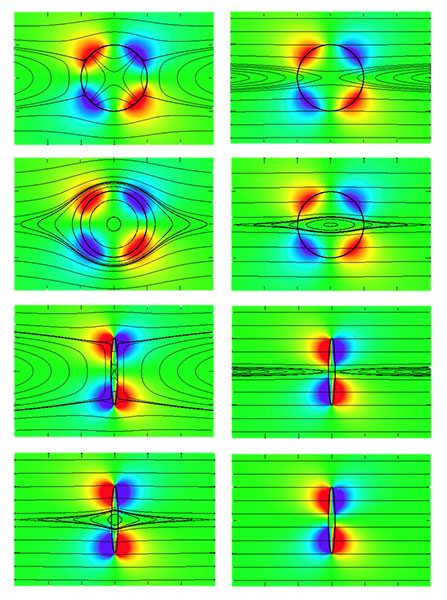
11. On the rotation of porous ellipsoids in simple shear flows
H. Masoud, H. A. Stone, and M. J. Shelley, Journal of Fluid Mechanics (Rapids) 733, R6 (2013) pdf
We study theoretically the dynamics of porous ellipsoids rotating in simple shear flows. We use the Brinkman–Debye–Bueche (BDB) model to simulate flow within and through particles and solve the coupled Stokes–BDB equations to calculate the overall flow field and the rotation rate of porous ellipsoids. Our results show that the permeability has little effect on the rotational behavior of particles and that Jeffery’s prediction of the angular velocity of impermeable ellipsoids in simple shear flows (Proc. R. Soc. Lond. A, vol. 102, 1922, pp. 161–179) remains an excellent approximation, if not an exact one, for porous ellipsoids. Employing an appropriate scaling, we also present approximate expressions for the torque exerted on ellipses and spheroids rotating in a quiescent fluid. Our findings can serve as the basis for developing a suspension theory for non-spherical porous particles or for understanding the orientational diffusion of permeable ellipses and spheroids.
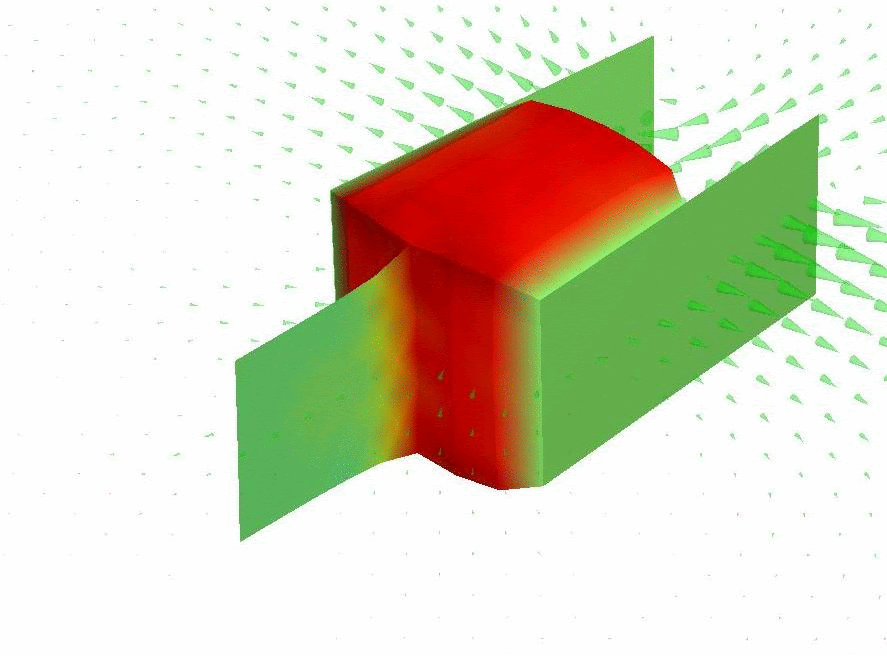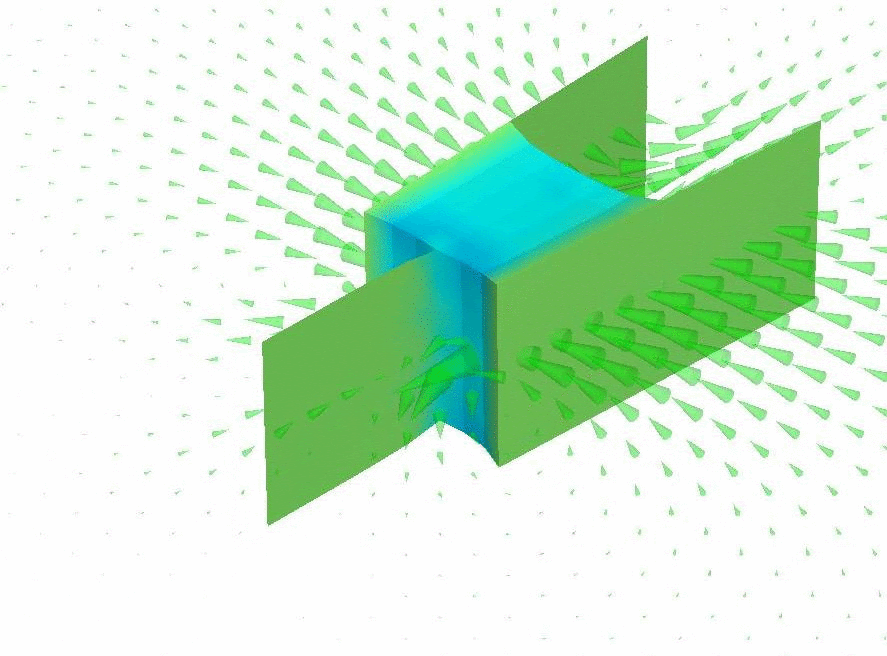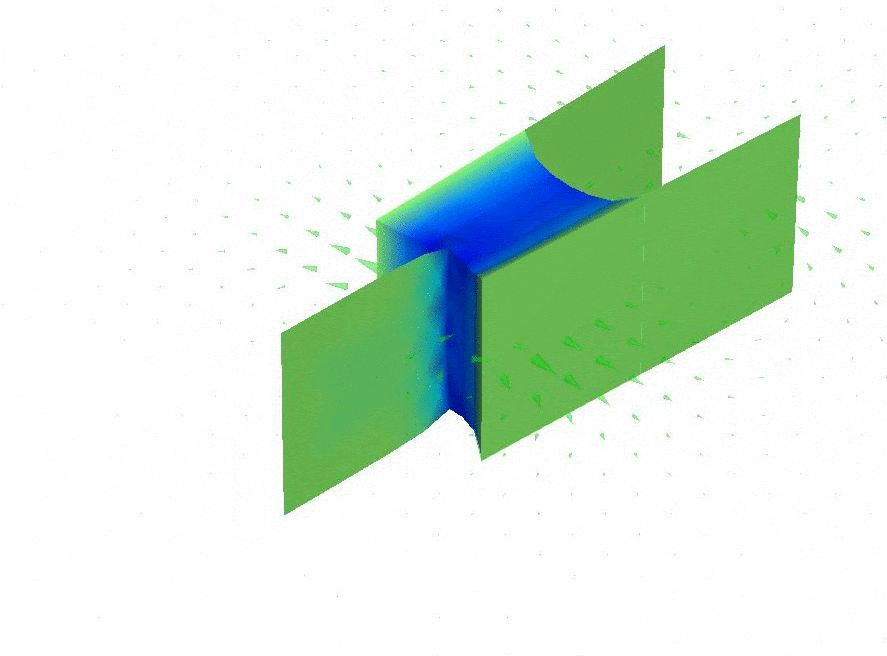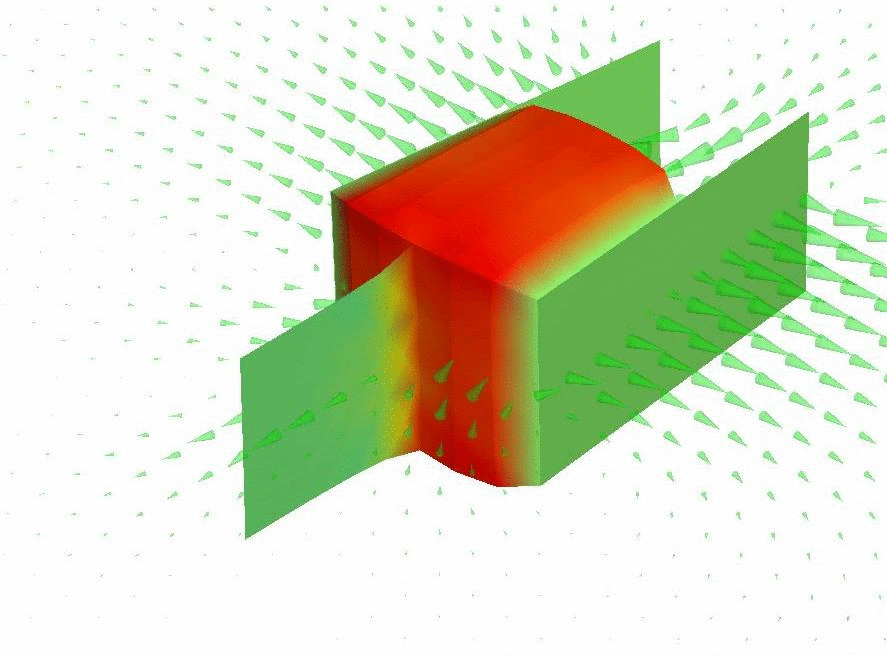
10. Designing maneuverable micro-swimmers actuated by responsive gel
H. Masoud, B. I. Bingham and A. Alexeev, Soft Matter 8, 8944 (2012) pdf
(Highlighted in Georgia Tech's Homepage, IEEE Computer Society News, ASME Nanotechnology Institute News, Soft Matter World Newsletter, Human Health and Science, Gizmag, Phys.Org, Georgia Tech College of Engineering, Science Daily, MedGadget, Communications of the ACM, and Newswise)
We use computational modeling to design a self-propelling micro-swimmer that can navigate in a low-Reynolds-number environment. Our simple swimmer consists of a responsive gel body with two propulsive flaps attached to its opposite sides and a stimuli-sensitive steering flap at the swimmer's front end. The responsive gel body undergoes periodic expansions and contractions, leading to a time-irreversible beating motion of the propulsive flaps, which propels the micro-swimmer. We examine the effects of body elasticity and flap geometry on the locomotion of the swimmer and show how they can be tailored to optimize the swimmer's propulsion. We also probe how the swimmer trajectory can be controllably changed using the steering flap that bends when exposed to an external stimulus. We demonstrate that the steering occurs due to two effects: steering flap bending and periodic beating. Furthermore, our simulations reveal that the turning direction can be regulated by changing the stimulus strength.
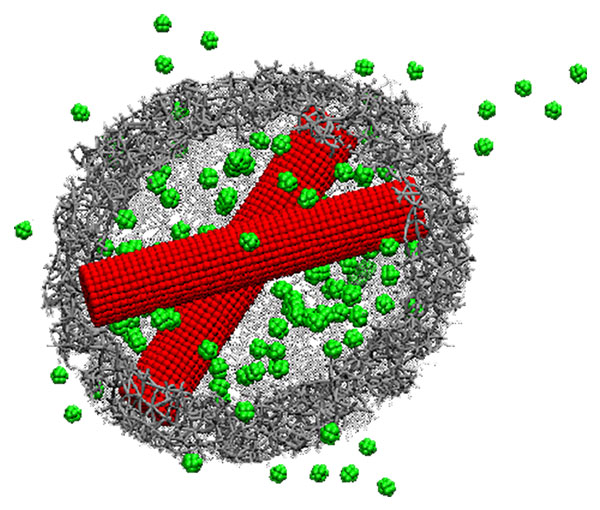
9. Controlled release of nanoparticles and macromolecules from responsive microgel capsules
H. Masoud and A. Alexeev, ACS Nano 6, 212 (2012) pdf
Using a mesoscale computational model, we probe the release of nanoparticles and linear macromolecules from hollow microgel capsules that swell and deswell in response to external stimuli. Our simulations reveal that responsive microcapsules can be effectively utilized for steady and pulsatile release of encapsulated solutes. Swollen gel capsules allow the steady, diffusive release of nanoparticles and polymer chains, whereas gel deswelling causes the burst-like discharge of solutes driven by an outward flow of the solvent enclosed within a shrinking capsule. We demonstrate that this hydrodynamic release can be regulated by introducing rigid microscopic rods in the capsule interior. Thus, our findings disclose an efficient approach for controlled release from stimuli-responsive microcarriers that could be useful for designing advanced drug delivery systems.
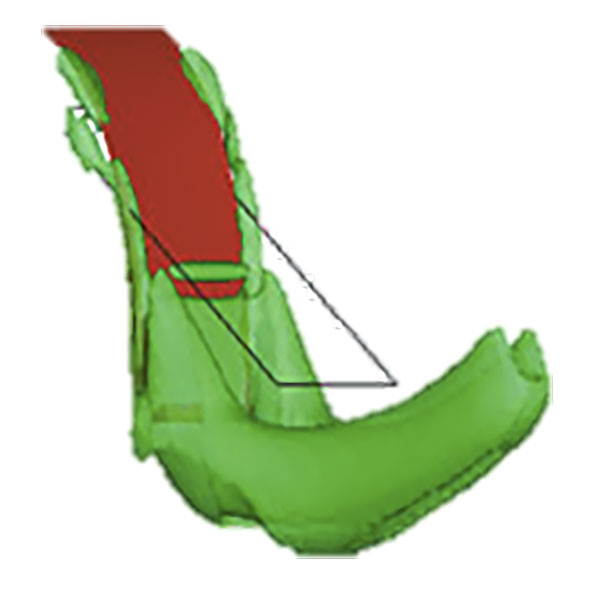
8. Efficient flapping flight using flexible wings oscillating at resonance
H. Masoud and A. Alexeev, In Natural Locomotion in Fluids and on Surfaces, Edited by S. Childress, A. E. Hosoi, W. W. Schultz, and Z. J. Wang, pp. 235-245, Springer, New York (2012) pdf
We use fully coupled three-dimensional computer simulations to examine the aerodynamics of elastic wings oscillating at resonance. Wings are modeled as planar elastic plates plunging sinusoidally at a low Reynolds number. The wings are tilted horizontally, thereby generating asymmetric flow patterns and non-zero net aerodynamic forces. Our simulations reveal that resonance oscillations of elastic wings drastically enhance aerodynamic lift, thrust, and efficiency. We show that flexible wings driven at resonance by a simple harmonic stroke generate lift comparable to that of small insects that employ significantly more complicated stroke kinematics. The results of our simulations point to the feasibility of using flexible resonant wings with a simple stroke for designing efficient microscale flying vehicles.
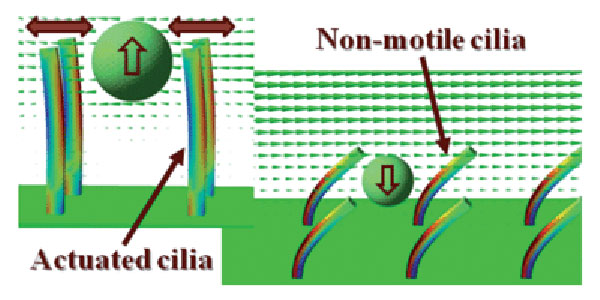
7. Harnessing synthetic cilia to regulate the motion of microparticles
H. Masoud and A. Alexeev, Soft Matter 7, 8702 (2011) pdf
(Invited "Highlight" article)
Functional synthetic cilia lining solid surfaces could potentially yield a unique approach for regulating transport processes at interfaces. We use computer simulations to probe how non-motile and actuated cilia can be harnessed to control the motion of microscopic particles suspended in a Newtonian fluid. We show that biomimetic cilia can be arranged to create hydrodynamic currents that can either direct particles towards the ciliated surface or expel them away, thereby modifying the effective interactions between solid surfaces and particulates. In addition to revealing new approaches for regulating microscale particle transport, our findings point to a new strategy for creating functional materials that employ active and responsive synthetic cilia.
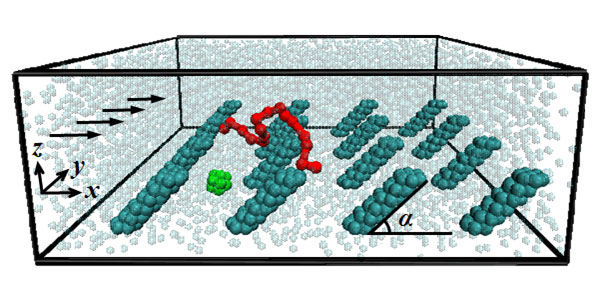
6. Selective control of surface properties using hydrodynamic interactions
H. Masoud and A. Alexeev, Chemical Communications 47, 472 (2011) pdf
(Invited paper for a special themed issue on Emerging Investigators)
(Highlighted in the Virtual Journal of Nanoscale Science & Technology 22, 25, 2010)
Using computational modeling, we design nano-structured surfaces that are able to selectively regulate interactions between microchannel walls and flowing colloid–polymer suspensions. Depending on the geometry of nanoscopic posts lining internal channel surfaces, suspended nanoparticles and polymeric chains can be either hydrodynamically attracted to channel walls or repelled to the bulk fluid.
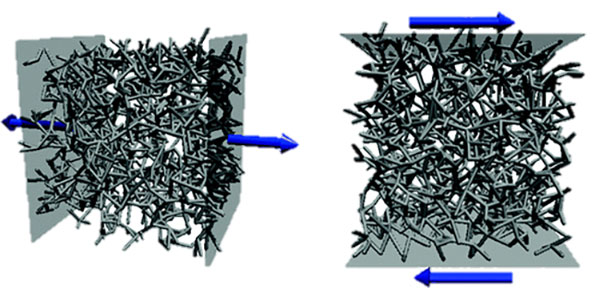
5. Permeability and diffusion through mechanically deformed random polymer networks
H. Masoud and A. Alexeev, Macromolecules 43, 10117 (2010) pdf
We develop a hybrid computational method to examine the permeation and hindered diffusion through mechanically loaded (anisotropic) polymer networks. We use the bond-bending lattice spring model to capture the micromechanics of random networks of interconnected filaments coupled with the dissipative particle dynamics to explicitly model the viscous fluid and diffusive objects. Our simulations reveal that the transport properties are independent of the network's internal organization and are solely a function of the network porosity and degree of anisotropy due to mechanical deformation. Furthermore, our results indicate that the network permeability under load can be estimated based on the network alignment that is characterized by a second-order orientation tensor. Our findings have implications for designing drug delivery agents, paper manufacturing, tissue engineering, and understanding the function of biological systems.

4. Resonance of flexible flapping wings at low Reynolds number
H. Masoud and A. Alexeev, Physical Review E 81, 056304 (2010) pdf
(Highlighted in Georgia Tech's Homepage, National Science Foundation News, US News & World Report, The Tech File, Georgia Tech College of Engineering, Innovations Report, ScienceMagNews, Gizmag, and Space Daily)
Using three-dimensional computer simulations, we examine the hovering aerodynamics of flexible planar wings oscillating at resonance. We model flexible wings as tilted elastic plates whose sinusoidal plunging motion is imposed at the plate root. Our simulations reveal that large-amplitude resonance oscillations of elastic wings drastically enhance aerodynamic lift and efficiency of low-Reynolds-number plunging. Driven by a simple sinusoidal stroke, flexible wings at resonance generate a hovering force comparable to that of small insects that employ very efficient but much more complicated stroke kinematics. Our results indicate the feasibility of using flexible wings driven by a simple harmonic stroke for designing efficient microscale flying machines.
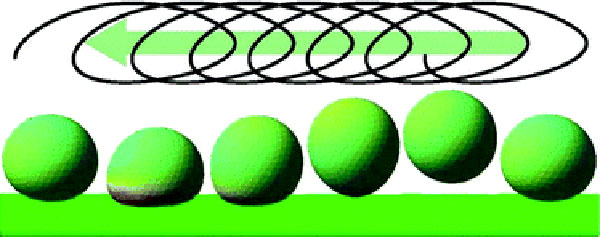
3. Modeling magnetic microcapsules that crawl in microchannels
H. Masoud and A. Alexeev, Soft Matter 6, 794 (2010) pdf
(Invited paper for a special issue on Emerging Themes in Soft Matter: Responsive and Active Soft Materials)
(Highlighted in the Virtual Journal of Nanoscale Science & Technology 21, 9, 2010)
Using computational modeling, we probe how to design microfluidic systems in which magnetic microcapsules could autonomously crawl along channel walls. The polymeric microcapsules are fluid-filled elastic shells with embedded superparamagnetic nanoparticles and, thereby, can be controlled by external magnetic fields. We show that when a magnetic force circulates normally to a sticky microchannel wall, capsules can propel in a steady, autonomous manner. The unidirectional capsule propulsion is facilitated by hydrodynamic interactions between the capsules and channel walls and is most effective when the magnitudes of magnetic and adhesive forces are equal to each other. Furthermore, the propulsion efficiency is greater for compliant capsules. Our findings could be useful for designing novel microfluidic devices where mobile magnetic microcapsules could be harnessed as microscale transport vehicles.

2. Analytical solution for Stokes flow inside an evaporating sessile drop: Spherical and cylindrical cap shapes
H. Masoud and J. D. Felske, Physics of Fluids 21, 042102 (2009) pdf
Exact analytical solutions are derived for the Stokes flows within evaporating sessile drops of spherical and cylindrical cap shapes. The results are valid for all contact angles. Solutions are obtained for arbitrary evaporative flux distributions along the free surface as long as the flux is bounded at the contact line. Specific results and computations are presented for evaporation corresponding to uniform flux and purely diffusive gas phase transport into an infinite ambient. Wetting and nonwetting contact angles are considered, and the flow patterns in each case are illustrated. For the spherical cap with evaporation controlled by vapor phase diffusion, when the contact angle lies in the range 0 ≤ θc < π/2, the mass flux of vapor becomes singular at the contact line. This condition requires modification when solving for the liquid-phase transport. Droplets in all of the above categories are considered for the following two cases: the contact lines are either pinned or free to move during evaporation. The present viscousflow behavior is compared to the inviscid flow behavior previously reported. It is seen that the streamlines for viscous flow lie farther from the substrate than the corresponding inviscid ones.
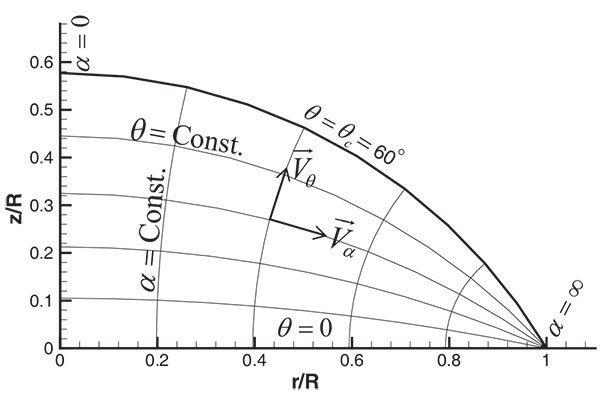
1. Analytical solution for inviscid flow inside an evaporating sessile drop
H. Masoud and J. D. Felske, Physical Review E 79, 016301 (2009) pdf
Inviscid flow within an evaporating sessile drop is analyzed. The field equation E2ψ = 0 is solved for the stream function. The exact analytical solution is obtained for arbitrary contact angle and distribution of evaporative flux along the free boundary. Specific results and computations are presented for evaporation corresponding to both uniform flux and purely diffusive gas-phase transport into an infinite ambient. Wetting and nonwetting contact angles are considered, with flow patterns in each case being illustrated. The limiting behaviors of small contact angles and droplets of hemispherical shape are treated. All of the above categories are considered for the cases of droplets whose contact lines are either pinned or free to move during evaporation.
